Answer:27th percentile
Step-by-step explanation:
Order the bid values in ascending order (smallest to largest)
60 85 95 105 120 145 155 175 190 215 235 240 260 285 315
Using the formula
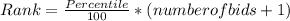
Rank of 105 is 4
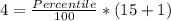
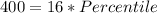
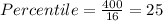
So the percentile of the bid value $105 is 25th percentile
But since 25th percentile is not among the options given, then 27th percentile will be the answer.
Going by the same formula, 27th percentile gives us the rank 4.05 which approximately is 4.