Question

A roll of material is (x + 2) metres wide. Annie buys (x + 3) metres of the material and Bronwyn buys 5 metres of the material.

a) Write an expression, in terms of x, for the area of each piece of material purchased.
b) If Annie has bought more material than Bronwyn, write an expression for how much more she has than Bronwyn.
c) Factorise and simplify this expression.
d) Find the width of the material if Annie has 5m^2 more than Bronwyn.
e) How much material does each person have? Explain.

Answer 1

Annie and Bronwyn buy two rectangles of material.

The width is common and it is x+2.

The height of Annie's rectangle is x+3, the height of Bronwyn is 5.

Since the area of a rectangle is the product of width and height, Annie's rectangle has an area of

`A = (x+2)(x+3)`

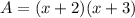
And Bronwyn's rectangle has an area of

`B = 5(x+2)`

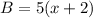
If Annie bought more than Bronwyn, the difference is

`A-B = (x+2)(x+3)-5(x+2) = (x+2)[(x+3)-5] = (x+2)(x-2)`

You can write this expression as you prefer:
`(x+2)(x-2)=x^2-4`

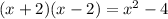
Question (d) represents the situation

`A=B+5 \implies (x+2)(x+3)=5(x+2)+5`

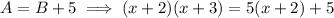
We can manipulate and solve this equation as follows:

`(x+2)(x+3)=5(x+2)+5 \iff x^2+5x+6=5x+15 \iff x^2-9=0 \iff x = \pm 3`

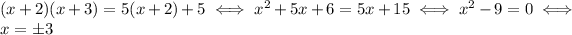
We can't accept the solution x = -3 because it would lead to negative dimensions. So, we have x=3, which implies the following dimensions and area:

`A = (x+2)(x+3) = 5\cdot 6 = 30`

`B = 5(x+2) = 5\cdot 5 = 25`

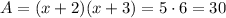
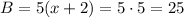
which indeed means that Annie has 5 squared meters more material than Bronwyn.