Answer:
the required equation is y = ( -2/3 )x -7
Explanation:
Given :-
the line passes through the point ( 0 , -7 )
)and slope of the line is ( -2/3 )
• To form an equation when one point and it's slope is given ,
• To form an equation when one point and it's slope is given , we use ;
• To form an equation when one point and it's slope is given , we use ;( y - y1 ) = m ( x - x1 )
• To form an equation when one point and it's slope is given , we use ;( y - y1 ) = m ( x - x1 )Where , x and y are variables
• To form an equation when one point and it's slope is given , we use ;( y - y1 ) = m ( x - x1 )Where , x and y are variablesand x1 and y1 are the points where the line meet !
• To form an equation when one point and it's slope is given , we use ;( y - y1 ) = m ( x - x1 )Where , x and y are variablesand x1 and y1 are the points where the line meet ! and m is slope of the tangent !!
y1 = -7
x1 = 0
So, putting all the values in the equation we get ,
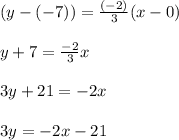
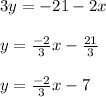
• Slope intercept form of the equation
• Slope intercept form of the equation y = mx + c
• Slope intercept form of the equation y = mx + c Where , m is slope of the line
• Slope intercept form of the equation y = mx + c Where , m is slope of the line and c is y intercept !!
[ also , we can directly put the value of c = -7 and m = ( -2/3 ) from given ]
Hence , here m = (-2/3 ) and c = -7
So, the required equation is y = ( -2/3 )x -7