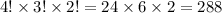Question

Suppose that you are organizing your texts for the semester at your desk consist-ing of 3 Computer Science, 2 Mathematics, and 2 Sociology textbooks. Assume alltextbooks are distinct. In how many ways can this be done ifa) the books can be arranged in any order?b) the Computer Science books must be together, but all other books can bearranged in any order?c) the Mathematics books and the Computer Science books must be together?

Answer 1

Answer : (a) 5040 b) 720 c) 288

Step-to step explanation:-

Given : There are 3 Computer Science, 2 Mathematics, and 2 Sociology textbooks.

Total books = 7

a) The number of ways the books can be arranged in any order is given by :-

`7!=7*6*5*4*3*2=5040`

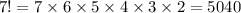
b) We consider all computer books as one thing, then, the total number of things to arrange = 1+2+2=5

Now, the number of ways to arrange books such that Computer Science books must be together, but all other books can be arranged in any order:-

`3!*5!\\\\=6*120=720`

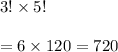
c) We consider all computer books as one thing and math book as one thing, then, the total number of things to arrange = 1+1+2=4

Now, the number of ways to arrange books such that Computer Science books must be together, but all other books can be arranged in any order:-

`4!* 3!*2!=24*6*2=288`