Question

A 35.1 kg wagon is towed up a hill inclined at 18.3 ◦ with respect to the horizontal. The tow rope is parallel to the incline and has a tension of 125 N in it. Assume that the wagon starts from rest at the bottom of the hill, and neglect friction. The acceleration of gravity is 9.8 m/s 2 . How fast is the wagon going after moving 75.4 m up the hill? Answer in units of m/s.

Answer 1

4.933m/s

Step-by-step explanation:

the wagon has a weight of 35.1kg*9.81m/s2 = 343.98N

of that weight 343.98N*sin(18.3)=108N are parallel to the hill and oposit tothe tension of the rope.

then, the force that is moving the wagon is 125N-108N=17N

F=m*a then 17N=35.1kg*a

a=0.4843 m/s2

we have two equations

`v=a.t\\x=v.t+(1)/(2) . a . t^(2)`

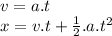
then

`x=a.t^(2) +(1)/(2). a t^(2) \\x=(3)/(2). a t^(2)`

`t=\sqrt{(2x)/(3a) }`

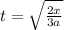
t=10.188s

`v=a.t`

v=4.933 m/s