Question

In the initial Cournot duopoly equilibrium, both firms have constant marginal costs, m, and no fixed costs, and there is a barrier to entry. Show what happens to the best-response function of firms if both firms now face a fixed cost of F Let market demand be p-a -bQ, where a and b are positive parameters with 2 firms. Let q1 and q2 be the amount produced by firm 1 and firm 2, respectively. Assuming it is optimal for the firm one to produce, its best-response function is I. (Properly format your expression using the tools in the palette. Hover over tools to see keyboard shortcuts. Eg., a subscript can be created with the- q1 = character.)

Answer 1

The best response functions are given by

`q_1=(a-m)/(2b)-(q_2)/(2)`

`q_2=(a-m)/(2b)-(q_1)/(2)`

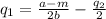
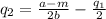
Step-by-step explanation:

Under no fixed costs the total costs is

`CT_i= mq_i`

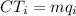
for i=1,2. The market demand is given by

`p=a-bQ`

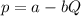
where
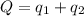`Q=q_1+q_2` is the total production

Firm 1 and 2 will maximize its own profits. Since this firms are symmetric the problems are too

`max\,\Pi_1=p=(a-b(q_1+q_2))q_1-mq_1`

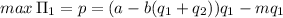
The first order conditions (take derivative of the profit with respect to
`q_1` are given by

`a-2 b q_1-b q_2-m=0`

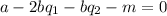
Then the best-response function for Firm 1 will be

`q_1=(a-m)/(2b)-(q_2)/(2)`

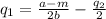
and the solution for Firm 2 would be the symmetric

`q_2=(a-m)/(2b)-(q_1)/(2)`

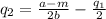
Now we can add fixed costs, so total costs now look

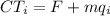`CT_i= F+mq_i` for i=1,2

the profit maximization problem for firm 1 looks now

`max\,\Pi_1=p=(a-b(q_1+q_2))q_1-F-mq_1`

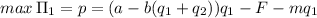
The first order conditions are given by

`a-2 b q_1-b q_2-m=0`

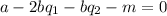
note that this equation is the same as in the absence of Fixed Costs. So the solutions would be the same. Fixed costs don't change the optimal level of production of these firms.

Note that Total Costs are given by fixed costs (F) and marginal costs (m) that depend on the production level of the firm

`CT_i=F+mq_i`

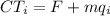
for i=1,2. The market demand is given by

`p=a-bQ`

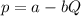
where
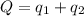`Q=q_1+q_2` is the total production, so it's the sum of each firms production

Firm 1 will maximize it's own profits

`max\,\Pi_1=p=(a-b(q_1+q_2))q_1-F-mq_1`

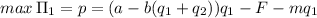
The first order conditions (take derivative of the profit with respect to
`q_1` are given by

`a-2 b q_1-b q_2-m=0`

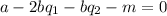
Then the best-response function for Firm 1 will be

`q_1=(a-m)/(2b)-(q_2)/(2)`

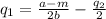
and the solution for Firm 2 would be symmetric.

Note that only marginal costs are relevant for getting the best-response function, so adding fixed costs (F) don't change the results

Step-by-step explanation: