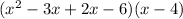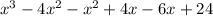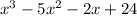Question

What is the polynomial function of least degree whose only zeros are -2, 3, and 4

Answer 1

`x^3-5x^2-2x+24`

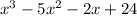
Explanation:

the polynomial function of least degree whose only zeros are -2, 3, and 4

Factors are -2,3 and 4

First we write the polynomial in factor form

If 'a' is a zero of the polynomial then (x-a) is a factor

Factors are -2,3 and 4

Polynomial is
`(x-(-2))(x-3)(x-4)`

`(x+2)(x-3)(x-4)`

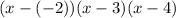
Now we multiply all the parenthesis

`(x+2)(x-3)(x-4)`

`(x^2-3x+2x-6)(x-4)`

`(x^2-x-6)(x-4)`

`x^3-4x^2-x^2+4x-6x+24`

`x^3-5x^2-2x+24`