Answer:
It is likely that C. Firm A will buy all of Firm B's pollution permits. Each one will cost between $100 and $200.
Step-by-step explanation:
- So two firms, A and B, each currently emit 100 tons of chemicals into the air, and from now on each one will require a pollution permit for each ton of pollution emitted into the air.
- Each firm gets 40 pollution permits, which it can either use or sell to the other firm. That means that if both firms choose to keep their respective 40 permits, they would still have to reduce the pollution by 60 tons (100 minus 40 is 60).
- It costs Firm A $200 for each ton of pollution that it eliminates before it is emitted into the air. Because it costs so much to eliminate a ton of pollution, it would make sense for Firm A to get as many pollution permits as possible, as long as they get them for less than $200 each.
- It costs Firm B $100 for each ton of pollution that it eliminates before it is emitted into the air. Since here it costs less to eliminate a ton of pollution, it would make sense for Firm B to sell as many pollution permits as possible, as long as they sell for higher than $100.
With that in mind, the outcome that makes the most sense would be Option C. Firm A will buy all of Firm B's pollution permits. Each one will cost between $100 and $200. This way both firms spend the least amount of money while at the same time pleasing the government.
To demonstrate it, let's do some actual calculations for each case.
Case A) Both firms will use their own pollution permits.
In this case, each firm will have to independently reduce their pollutants by 60 tons, as noted before. That represents a high cost, as we will now determine:
For Firm A, the cost would be
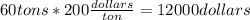
For Firm B, the cost would be
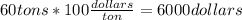
Case B) Firm A will buy some of Firm B's pollution permits. Each one will cost less than $100.
Since Firm B could spend $100 to reduce a ton of pollution, it wouldn't sell its pollution permits for less than $100 each: If Firm B sold its pollution permits for less than $100 each, it would have to reduce even more tons of pollutants (spending $100 for each one), and would end up losing money! Let's say it sold 10 pollution permits for $90 each, so it would have to reduce 70 tons of pollutants instead of 60. Its total cost would be:
Cost for Firm B (Case B):
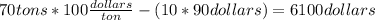
Which is higher than the cost calculated for Firm B in Case A, so it's not worth it.
Case D) Firm B will buy all of Firm A's pollution permits. Each one will cost between $100 and $200.
This is a similar case than Case B, in the sense that since it costs Firm A so much to reduce a ton of pollutant ($200 for each one), it wouldn't sell its pollution permits for less than $200 each, or it would end up losing money as well. Let's say Firm A sold all of its 40 pollution permits for $150 each, and so it would have to reduce 100 tons of pollutants instead of 60. Its total cost would be:
Cost for Firm A (Case D):
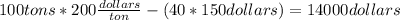
Which is higher than the cost calculated for Firm A in Case A, so it's not worth it.
Finally, Case C) Firm A will buy all of Firm B's pollution permits. Each one will cost between $100 and $200.
As mentioned before, this one makes the most sense because both firms would spend the least amount of money. Let's determine the total costs for each one, knowing that:
- Firm A would buy 40 pollutant permits from Firm B, for (let's say) $150 each.
- Firm A would still need to reduce 20 tons of pollutants. And
- Firm B would have to reduce 100 tons of pollutants, instead of 60.
Cost for Firm A (Case C):
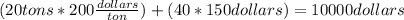
Which is less than the $12000 Cost calculated in Case A.
Cost for Firm B (Case C):
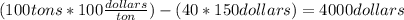
Which is less than the $6000 Cost calculated in Case A.
Since both firms each spend $2000 less in Case C than in case A, it would make sense for them to follow this option.