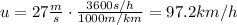Question

A car is moving at a constant velocity when it is involved in a collision. The car comes to rest after 0.450 s with an average acceleration of 60.0 m/s2 in the direction opposite that of the car's velocity. What was the speed, in km/h, of the car before the collision?

Answer 1

97.2 km/h

Step-by-step explanation:

We can solve the problem by using the following SUVAT equation:

`a=(v-u)/(t)`

where

v = 0 is the final velocity of the car

u is the initial velocity

a = -60.0 m/s^2 is the acceleration (negative, as it is in the opposite direction as the velocity)

t = 0.450 s is the time it takes for the car to comes to a stop

Solving for u, we find

`u=v-at=0-(-60.0)(0.450)=27 m/s`

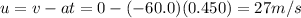
In order to convert it into km/h, let's keep in mind that:

1 km = 1000 m

1 h = 3600 s

So we find:

`u=27 (m)/(s) \cdot (3600 s/h)/(1000 m/km)=97.2 km/h`