Question

Which represents the solution to the above absolute value equation? 2|x+2|-1=5

Answer 1

Answer: C, x = 1 or x = -5

Explanation:

Answer 2

C. x=1 or x=-5

Explanation:

1. First, we have to get the absolute value alone.

2|x+2|-1=5

2. Add 1 to both sides.

2|x+2|-1 +1 = 5 +1. The -1 and +1 will cancel each other out.

You will get 2|x+2|=6.

3. Divide 2 by both sides.

`(2|x+2|)/(2)` =
`(6)/(2)`

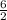
The division of 2 and the multiplication of 2 on the side of the absolute value will also cancel each other out.

You will get |x+2|=3.

4. Next, we will split this absolute value equation into two equations because we don't know if the variable of x that's inside of the absolute value is negative or not.

x+2=3 or x+2=-3.

5. Subtract 2 from all sides.

x+2 -2 =3 or x+2 -2 =-3

You will get x=1 or x=-5.

x=1 or x=-5 is your final answer.