Answer:
In order for the second plan to be preferable, a greater amount of 249.6 minutes would have to be used in one month.
Explanation:
Let
x -----> the number of minutes in a month
y ----> the charge monthly
we know that
First Plan
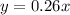 ----> equation A
----> equation A
Second Plan
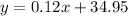 ----> equation B
----> equation B
Equate the equations A and B and solve for x


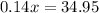
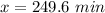
so
For
 ----> is preferable the first plan
----> is preferable the first plan
For
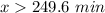 ----> is preferable the second plan
----> is preferable the second plan
therefore
In order for the second plan to be preferable, a greater amount of 249.6 minutes would have to be used in one month.