Question

A bond with a face value of $1,000 and 15 years remaining until maturity pays a coupon rate of 10% semi-annually. Calculate its yield to maturity if it is priced at $1,100.

Answer 1

Ans. YTM=8.9805%

Explanation:

Hi, first we need to determine the coupon for every semester and construct a table in order to use the "IRR" function of microsoft excel. Everything should look like this.

face value $1.000

Price $1.100

time 15 years to maturity

coupon 10% annual

5% semi annual

coupon(semi anual) $50

0 -$1.100

1 $50

2 $50

3 $50

4 $50

5 $50

6 $50

7 $50

8 $50

9 $50

10 $50

11 $50

12 $50

13 $50

14 $50

15 $50

16 $50

17 $50

18 $50

19 $50

20 $50

21 $50

22 $50

23 $50

24 $50

25 $50

26 $50

27 $50

28 $50

29 $50

30 $1.050

There are 30 periods since the coupon is to be paid semi annually, the coupon is calculated as follows.

`Coupon=FaceValue((Coupon Rate(annual))/(2) )=1000(0.1)/(2) =50`

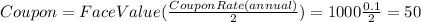
Notice that the value of period 0 is -1100, corresponds to the amount of money paid for the bond and is negative because, well, we paid for it. This is very important because when you use the IRR formula of MS Excel, it requires at least 1 change of sign to work properly.

So the answer at this point is 4.3937% but remember that the cash flow took place in a semi-annual fashion, therefore, we have to make it annual, this is the math to that.

`YTM(annual)=(1+YTM(semi annual))^(2) -1=(1+0.043937)^(2) -1=8.9805% annual`

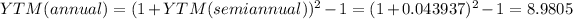
Best of luck.