Question

A small software corporation borrowed $ 250,(000) to expand its software line. The corporation borrowed some of the money at 9%, some at 10%, and some at 12%. Use a system of equations to determine how much was borrowed at each rate if the annual interest rate was $26,000 and the amount borrowed at 10% was 2.5 times the amount borrowed at 9%.

Answer 1

$50,000 was borrowed at 9%.

$125,000 was borrowed at 10%.

$75,000 was borrowed at 12%.

Explanation:

Let amount borrowed at 9% be x

Let amount borrowed at 10% be y

Let amount borrowed at 12% be z

Now equations form:

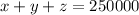`x+y+z=250000` ....(1)

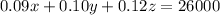`0.09x+0.10y+0.12z=26000` ....(2)

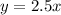`y=2.5x` ..... (3)

Substituting the value of y from (3) in (1) and (2)

`x+2.5x+z=250000`

=>
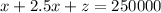
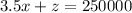`3.5x+z=250000` ......(4)

`0.09x+0.10(2.5x)+0.12z=26000`

=>
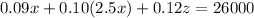
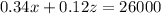`0.34x+0.12z=26000` ....(5)

Multiplying (4) by 0.12 and subtracting (5) from it, we get

`0.42x+0.12z=30000` -
`0.34x+0.12z=26000`

`0.42x-0.34x=30000-26000`

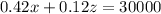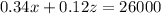
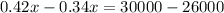
0.08x=4000

x = $50000

As y = 2.5x

So,
`y=2.5*50000=125000`

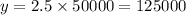
y = $125000

And as x+y+z=250000

=>
`z=250000-50000-125000`

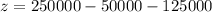
z = $75000

Therefore, $50,000 was borrowed at 9%.

$125,000 was borrowed at 10%.

$75,000 was borrowed at 12%.