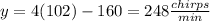Answer:
a)
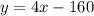
b)
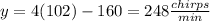
Explanation:
We know that theres a linear relationship between the rate of the chirping of crickets and the air temperature.
The equation of a line is:

So, let's name our variables
x= Temperature
y=Rate of the chirping
First of all, we need to find the slope with the two given points
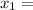 60ºF ,
60ºF ,
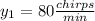
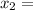 80ºF,
80ºF,
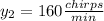
By,
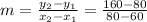
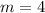
Now, the equation between the air temperature and the number of chirps is:
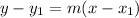
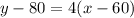
Solving for y,
a)
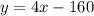
b) To calculate the rate at which the crickets chirp when the temperature is 102 ºF we need to evaluate y(102)