Answer:
FIRST QUESTION
It will pay the bill after 7.45 = rounding 8 months, being the last payment less than 5,000
The total interest for the period will be 2,263.04
SECOND QUESTION The rate will be 6%
Explanation:
for the first part, we should calculate the time it takes to an ordinary annuity of 5,000 to have a present value of 35,000 at discount rate of 18%

C 5,000
time n
rate 0.015
PV $35,000.0000

We work out the formula:
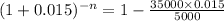
We solve the right side of th formula
and then apply logarithmics properties:
![-n= \frac{log0.895}{log(1+0.015)]()
-n = -7.450765527
n = 7.45
It will pay the bill after 8 months.
Total Interest: we will build the loan schedule:
Bill Interest Cuota&Amortization
1 35000 525& 5000 4475
2 30525 457.88 5000 4542.12
3 25982.88 389.74 5000 4610.26
4 21372.62 320.59 5000 4679.41
5 16693.21 250.4 5000 4749.6
6 11943.61 179.15 5000 4820.85
7 7122.76 106.84 5000 4893.16
8 2229.6 33.44 2263.04 2229.6
Total 2263.04 37263.04 35000
The total interest for the period will be 2,263.04
Second question we will solve for the rate at which a capital of 3,000 returns 5,372.54 after 10 years
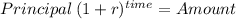
Principal 30,00.00
Amount = 5,372.54
time 10.00
rate ?
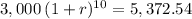
![r = \sqrt[10]{5,372.54/3,000} - 1](https://img.qammunity.org/2020/formulas/mathematics/college/ptbho4vpzcdtaog0hffezak56w1xa6sp10.png)
r = 0.059999939 = 0.06 = 6%