Question

An electron has a velocity of 3.2 x 10^6 m/s. What is its’ momentum? (b) What is its’ wavelength? (c) What other objects/materials have this space/size? (d) Assuming that we can measure the velocity to an accuracy of 10%. Use the Heisenberg uncertainty principle to calculate the uncertainty in the position.

Answer 1

P = 2.91*10^{-24} kg m/s

`\lambda = 2.73 *10^(-10) m`

size of atom hat lie in range of 1 to 5 Angstrom

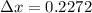`\Delta x = 0.2272` Angstrom

Step-by-step explanation:

A) MOMENTUM

p = mv

where m is mass of electron

so momentum p can be calculated as

p = 9.11*10^{-31} *3.2*10^{6}

P = 2.91*10^{-24} kg m/s

b) wavelength

`\lambda = (h)/(mv)`

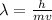
where h is plank constant

so
`\lambda = (6.626*10^(-34))/(2.91*10^(-24))`

`\lambda = 2.73 *10^(-10) m`

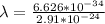
c) size of atom hat lie in range of 1 to 5 Angstrom

d) from the information given in the question we have

`(\Delta v)/(v) = 0.1`

`\Delta v = 0.1 v`

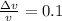
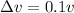
we know that

`\Delta p *\Delta x = (h)/(4\pi)`

`m \Delta v \Delta x =(h)/(4\pi)`

`\Delta x = (h)/(m \Delta v)`

`\Delta x  = (2.272)/(0.1)` [
`\Delta v = 0.1 v`]

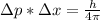
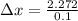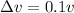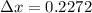`\Delta x = 0.2272` Angstrom