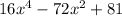Answer:
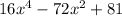
Explanation:
First, lets think of this binomial as
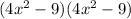
To do this, we will have to use the FOIL method. This means that we multiply the First terms, Outside terms, Inside terms, and Last terms.
The first terms are both
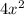 , so lets multiply these first
, so lets multiply these first

Next lets multiply the outside terms, which are
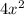 and
and


Now the inside terms, which are also
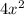 and
and


Lastly, the last terms, which are both


Now we have to add each of these together.
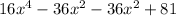
The last step is to combine like terms
This gives us