Question

For one toss of a certain coin, the probability that the outcome is heads is 0.6. If this coin is tossed 5 times, which of the following is the probability that the outcome will be heads at least 4 times?

Answer 1

The probability is 0.33696

Explanation:

The probability that the outcome will be heads x times is calculated using the following equation:

`P(x) = nCx*p^(x) *(1-p)^(n-x)`

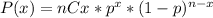
nCx is calculated as:

`nCx=(n!)/(x!(n-x)!)`

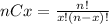
This apply for variables that follows a binomial distribution. In which we have n independent and identical events with two possibles results: success and fail with a probability p and 1-p respectively.

So, In this case, n is equal to 5, and p is equal to 0.6 because we are going to call success the event in which the outcome of the coin is head.

Then, the probability that the outcome will be heads at least 4 times is calculated as:

P = P(4) + P(5)

Where P(4) is:

`P(4) = 5C4*0.6^(4) *(1-0.6)^(5-4)`

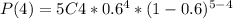
P(4)=0.2592

And P(5) is:

`P(5) = 5C5*0.6^(5) *(1-0.6)^(5-5)`

P(4)=0.07776

Finally, the probability is:

P = 0.2592 + 0.07776

P = 0.33696