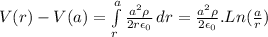Question

Calculate the potential of a infinitely long cylinder of radius a which has constant charge density p everywhere in space. Your answer should be in cylindrical coordinates. b) How does the potential change if a cylinder of radius b < a is hollowed out of the middle so that the axis through their centers coincide. Your answer should be in cylindrical coordinates. c) What is the electric field created in the above scenarios, use the same coordinates as the corresponding scenario.

Answer 1

Step-by-step explanation:

I will use the Gauss's Law to find the field for both cases, after this I'll calculate the potential.

`\int\ E.} \, dS = (Qin)/(\epsilon )`

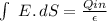
the surface S is an "infinite long" cylinderr of radio r, the normal vector only has radial coordinates.

E(r)=E(r)r /*The field, based on cylindrical symmetry, only depends of the radius, and only has radial coordinate*/

`\int\ {E.} \, dS = E\int\ dS=E.S=E2\pi rL`

`Qin=\int\, dq', \rho=(dq')/(dVol'), Qin=\int\limits_ V {\rho} \, dVol'`

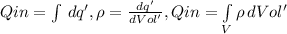
if r<a
`E(r)=(r\rho)/(2 \epsilon_(0) )` with radial versor

if r>=a the gaussian surface encloses all the charge, so

Qin=p.Vol=
`\rho.\pi .a^(2).L`

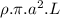
then:
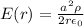`E(r)=(a^(2)\rho)/(2r\epsilon_(0) )` with radial versor.

In the case when the cylinder has a hole, the field will be null there (because there is not charge inside the gaussian). to calculate the field I can suppose that in the hole I have an charge density -ρ, to cancel with the other.

Potential:

V(r)-V(a)=
`\int\limits^a_r {E(r)} \, dr`

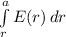
if r<a

`V(r)-V(a)=\int\limits^a_r {(r\rho)/(2\epsilon_(0) ) } \, dr = (\rho.(a^(2)-r^(2)) )/(4\epsilon_(0))`

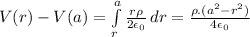
if r>a

`V(r)-V(a)=\int\limits^a_r {(a^(2)\rho)/(2r\epsilon_(0) ) } \, dr = (a^(2)\rho)/(2\epsilon_(0)).Ln((a)/(r))`