Answer:
(a). (i). The reactants are
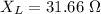 .
.
(II). The inductance of the circuit is 0.1583 Henry.
(b). The resonant angular frequency is 229.4 rad/s.
Step-by-step explanation:
Given that,
Capacitor = 120.0 μC
Frequency = 200.0 rad/s
Impedance = 100.0 -10j
(I). We need to calculate the
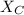
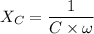
Put the value into the formula

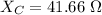
(II). We know that,
Formula of impedance is
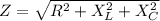 ...(I)
...(I)
Given equation of impedance is
 ...(II)
...(II)
On Comparing of equation (I) and (II)
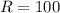
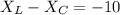
Now, put the value of
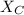
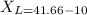

We need to calculate the inductance
Using formula of inductance
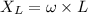
Put the value into the formula
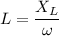
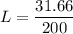
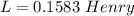
(b). We need to calculate the resonant angular frequency
Using formula of the resonant angular frequency
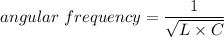
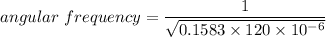
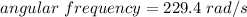
Hence, (a). (i). The reactants are
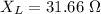 .
.
(II). The inductance of the circuit is 0.1583 Henry.
(b). The resonant angular frequency is 229.4 rad/s.