Question

Suppose that after 10 years of service, 40% of computers have problems with motherboards (MB), 30% have problems with hard drives (HD), and 15% have problems with both MB and HD. What is the probability that a 10-year old computer still has fully functioning MB and HD?

Answer 1

The probability that a 10-year old computer still has a functioning motherboard (MB) and hard drive (HD) is 45%, calculated using the complement rule based on the given probabilities of individual and joint problems.

Step-by-step explanation:

To find the probability that a 10-year old computer still has fully functioning motherboard (MB) and hard drive (HD), we use the complement rule. According to the provided data, 40% of computers have problems with MB, 30% have problems with HD, and 15% have problems with both MB and HD. We first need to find the probability that a computer has either MB or HD problems, or both, using the formula P(A or B) = P(A) + P(B) - P(A and B).

In this case, P(MB or HD problems) = P(MB problems) + P(HD problems) - P(both problems) = 0.40 + 0.30 - 0.15 = 0.55. This means that 55% of computers have either MB or HD problems or both after 10 years.

The probability that a computer has no MB or HD problems is the complement of this probability, which is 1 - P(MB or HD problems) = 1 - 0.55 = 0.45 or 45%.

Therefore, the probability that a 10-year old computer still has fully functioning MB and HD is 45%.

Answer 2

The probability that a 10-year old computer still has fully functioning MB and HD is 45%

Step-by-step explanation:

The probability of the union of two events, is equal to the sum of the individual probabilities of the two events, minus the probability of the intersection event.

That means that the probability of have a fully functional computer (PFC) is equal to the sum of probability of have a computer with a functional motherboards (PFMB) plus the probability of have a computer with a functional hard drives (PFHD), minus the probability of have a computer with both motherboards and hard drives functional (PBMH).

PFC = PFMB+PFHD-PBMH

• Probability of functional motherboards (PFMB)

PFMB = 1 - probability of having problems with motherboards = 1 - 40 %

PFMB = 1 -
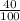`(40)/(100)` = 1 -
`(4)/(10)` =
`(6)/(10)`

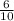
• Probability of functional hard drive (PFHD)

PFHD = 1 - probability of having problems with hard drive = 1 - 30 %

PFHD = 1 -
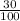`(30)/(100)` = 1 -
`(3)/(10)` =
`(7)/(10)`

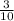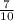
• Probability of functional both (PFMH)

PFMH = 1 - probability of having problems with both = 1 - 15 %

PFMH = 1 -
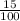`(15)/(100)` = 1 -
`(3)/(20)` =
`(17)/(20)`

PFC =
`(6)/(10)` +
`(7)/(10)` -
`(17)/(20)`

PFC =
`(9)/(20)`

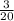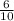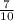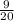
PFC = 0.45

Multiply by 100 to get in in %

The probability that a 10-year old computer still has fully functioning MB and HD is 45%