Step-by-step explanation:
The given data is as follows.
 (NaCl) =
(NaCl) =

 (H-O=C-ONO) =
(H-O=C-ONO) =

 (HCl) =
(HCl) =
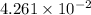
Conductivity of monobasic acid is
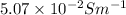
Concentration = 0.01
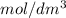
Therefore, molar conductivity (
 ) of monobasic acid is calculated as follows.
) of monobasic acid is calculated as follows.

=
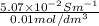
=
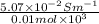
=
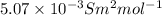
Also,
 =
=
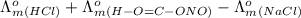
=
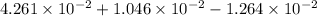
=
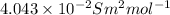
Relation between degree of dissociation and molar conductivity is as follows.

=

= 0.1254
Whereas relation between acid dissociation constant and degree of dissociation is as follows.
K =
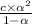
Putting the values into the above formula we get the following.
K =
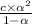
=
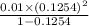
=
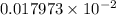
=

Hence, the acid dissociation constant is
 .
.
Also, relation between
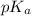 and
and
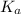 is as follows.
is as follows.
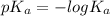
=
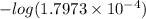
= 3.7454
Therefore, value of
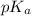 is 3.7454.
is 3.7454.