Answer:
At any time t, her altitud will be given by the following law:

Step-by-step explanation:
We can consider the gymnast as a point particle in the position of her center of mass. The law of movement will be:

where g is gravity's acceleration which we can take as
 . We add 0 in the last member just to note that at
. We add 0 in the last member just to note that at
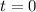 the vertical position is 0. If we knew that she was moving at
the vertical position is 0. If we knew that she was moving at
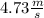 at
at
 , we would have needed to put 1m in the place of 0.
, we would have needed to put 1m in the place of 0.