Question

Find x
A. 22√2
B. 22
C. 11√3/3
D. 11√3

Answer 1

A. 22√2.

Explanation:

The diagonal of the small triangle = 11√2 ( because it is a 45-45-90 triangle).

cos 60 = 11√2 / x

x = 11√2 / cos 60

= 11√2 / 1/2

= 22√2.

Answer 2

`22√(2)`

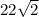
Explanation:

First we gave a name to the side that is common for both triangles, can be Y for example.

Using trigonometric functions we can make a relationship between the two angles that we have and the magnitud of the side.

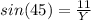`sin(45)=(11)/(Y)  \\` , Because sin(x)= op/hip

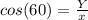`cos(60)=(Y)/(x)` , Because con(x)=ad/hip

Having this equations, we can solve the system and find x

`Y=(11)/(sin(45)) \\Y=cos(60)*x`

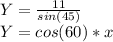
we equal Y and solve

`(11)/(sin(45))=cos(60)*x\\ x=(11)/(sin(45)*cos(60)) \\x=(11)/((√(2) )/(2) *(1)/(2) ) \\x=(11)/((√(2) )/(4) ) \\x=(44)/(√(2) )\\`

Then we rationalize

`x=22√(2)`

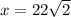
Hope you like it!