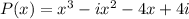Answer:
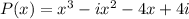
Explanation:
We have to find the polynomial of lowest degree with lead coefficient 1 and roots i, -2 and 2.
A polynomial can be written as:
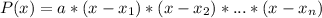
Where
 is the lead coefficient. And
is the lead coefficient. And
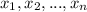 are the roots of the polynomial.
are the roots of the polynomial.
Then we have
 and,
and,
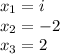
We can write the polynomial as:
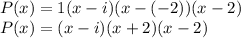
You can apply squared binomial to
 :
:
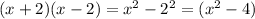
Then,
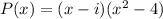 apply distributive property:
apply distributive property:
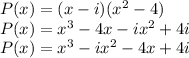
The the polynomial of lowest degree with leaf coefficient 1 and roots i, -2 and 2 is: