Question

The electric field of a plane standing electromagnetic wave in a vacuum is given by Ey- Eosin(kx)cos(ot). What is the corresponding expression for the magnetic field? Bz =-cos(kx)sin(at) B B cos(kox)cos(ot) Bz-sin(kx)cos(ot) Bz cos(kx)sin(at) E)Bz-sin(kx)cos(at) C) Eo

Answer 1

`B=-(E_o)/(c)cos(kx)sin(\omega t)\ k`

Step-by-step explanation:

The electric field of a plane standing electromagnetic wave in a vacuum is given by :

`E_y=E_o\ sin(kx)cos(\omega t)`

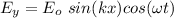
We need to find the corresponding expression for the magnetic field. According to equation of Maxwell's :

`\bigtriangledown * E=-(\partial B)/(\partial t)`

`\bigtriangledown * E=\begin{vmatrix}i & j & k\\ (\partial)/(\partial x) & (\partial )/(\partial y) & (\partial)/(\partial z)\\ 0& E_o\ sin(kx)cos(\omega t) & 0 \end{vmatrix}`

`\bigtriangledown * E=k[E_ok\ cos(kx)cos(\omega t)]=-(\partial B)/(\partial t)`

`B=\int\limits {kE_ok\ cos(kx)cos(\omega t).dt}`

`B=-(E_ok)/(\omega)cos(kx)sin(\omega t)`

Since,
`\omega=ck`

`B=-(E_o)/(c)cos(kx)sin(\omega t)\ k`

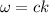
So, the corresponding expression for the magnetic field is
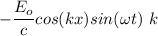`-(E_o)/(c)cos(kx)sin(\omega t)\ k`. Hence, this is the required solution.