Question

A silver wire with resistivity 1.59 × 10-8 Ω-m carries a current density of 4.0 A/mm2, What is the magnitude of the electric field inside the wire? 10 A) 0.064 V/m B) 2.5 V/m C) 0.10 V/m D) 0.040 V/m

Answer 1

Electric field, E = 0.064 V/m

Step-by-step explanation:

It is given that,

Resistivity of silver wire,
`\rho=1.59* 10^(-8)\ \Omega-m`

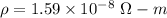
Current density of the wire,
`J=4\ A/mm^2=4* 10^6\ A/m^2`

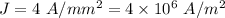
We need to find the magnitude of the electric field inside the wire. The relationship between electric field and the current density is given by :

`E=J* \rho`

`E=4* 10^6* 1.59* 10^(-8)`

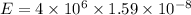
E = 0.0636 V/m

or

E = 0.064 V/m

So, the magnitude of electric field inside the wire is 0.064 V/m. Hence, this is the required solution.