Question

Commercial aircraft used for flying in instrument conditions are required to have two independent radios instead of one. Assume that for a typical​ flight, the probability of a radio failure is 0.00260.0026. What is the probability that a particular flight will be safe with at least one working​ radio? Why does the usual rounding rule of three significant digits not work​ here? Is this probability high enough to ensure flight​ safety?

Answer 1

We are given that Commercial aircraft used for flying in instrument conditions are required to have two independent radios instead of one.

The probability of failure of one radio = 0.0026

So, the probability of failure of both radio =
`0.0026 * 0.0026=0.00000676`

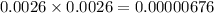
Now we are supposed to find the probability that a particular flight will be safe with at least one working​ radio

A) the probability that a particular flight will be safe with at least one working​ radio = 1- 0.00000676=0.99999324

B)The usual rounding rule of three significant digits not work​ here because usual rounding rule of three significant digits makes it 1 which means that both radios are working properly.

C)Yes,this probability is high enough to ensure flight​ safety because it is close to 1