Answer:
Part a)98 km/h
Part b) 93.5 km/h
Part c) 95.7 km/h
Step-by-step explanation:
A) For the trip from Antonio to Houston we have
Let the total time in which the trip is completed be 't'
Thus the distance covered with a speed of 77 km/h equals
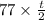
Similarly the distance covered with a speed of 119 km/h equals
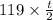
Now by definition of average speed we have
Average Speed =
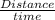
Applying values we get
Average Speed for trip 1 =
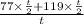
Thus average speed equals
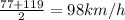
B)For the trip from Houston Antonio to we have
Let the total distance of the trip be 'd'
Time required to complete half the distance at a speed of 77 km/h equals
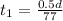
Time required to complete remaining half the distance at a speed of 119 km/h equals
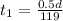
Now by definition of average speed we have
Average Speed =
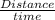
Applying values we get
Average Speed for trip 1 =
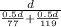
Thus average speed equals

C) For the entire trip we have
Average Speed =
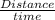
Applying values we get
Average Speed for both the trips =
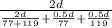
Thus average speed for both the trips equals 95.7 km/h