Question

A car moves along an x axis through a distance of 910 m, starting at rest (at x = 0) and ending at rest (at x = 910 m). Through the first 1/4 of that distance, its acceleration is +7.20 m/s2. Through the next 3/4 of that distance, its acceleration is -2.40 m/s2. What are (a) its travel time through the 910 m and (b) its maximum speed?

Answer 1

a)Total time = 31.6 s

b)The maximum speed of car will be 56.88 m/s

Step-by-step explanation:

Given that

Initial velocity u = 0

x= 910 m

y= 1/4 of 910 m= 227.5 m

`a=7.2\ m/s^2`

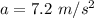
z= 3/4 of 910 = 682.5 m

`a= - 2.4\ m/s^2`

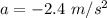
Time take to cover 227.5 m:

We know that

`s=ut+(1)/(2)at^2`

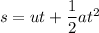
Here initial velocity u= 0 because it start from rest

`227.5=0+(1)/(2)* 7.2* t^2`

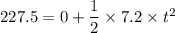
So t = 7.9 sec

So the velocity after 7.9 s

V = ut +at

V= 0+ 7.2 x 7.9

V= 56.88 m/s

Time take to cover 682.5 m:

The initial velocity for V= 56.88 m/s

The final speed of car will be zero because

So V'= V - at

0=56.88 - 2.4 x t

t=23.7 s

So the total time to cover distance 910 m =23.7 + 7.9 s

Total time = 31.6 s

The maximum speed of car will be 56.88 m/s