Question

two byciclists, starting at the same place, are riding toward the same campground by two different routes. One cyclist rides 1080 m due east and then turns due north and travels another 1430 m before reacing the campground. The second cyclist starts out by heading due north for 1950 m and then turns and heads directly toward the campground. (a) at the turning point, how far is the second cyclist from the campground

Answer 1

Answer: 1198 m

Hi, in the image you can see the routes taken by both cyclists.

Cyclist 1 route is AB then BC

Cyclist 2 route is AD then DC

C is the campground

You need to calculate DC (D is the turning point). You need to find the distance DX, and use Pythagoras theorem with the triangle DXC

You know that AB = 1080m, BC = 1430m, AD = 1950m

Then DX = 1950m - 1430m = 520m

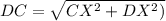`DC = √(CX^2 + DX^2))` = 1198m