Question

Lines a and b are cut by transversal f. At the intersection of lines f and a, the top left angle is 96 degrees. At the intersection of lines b and f, the bottom right angle is (6 x minus 36) degrees.

What must be the value of x so that lines a and b are parallel lines cut by transversal f?

10
20
22
32

Answer 1

Option C.

Explanation:

It is given that lines a and b are cut by transversal f.

If a transversal line intersect two parallel lines, then the alternate exterior angles are same.

We need to find the value of x so that lines a and b are parallel lines cut by transversal f.

So, equate both alternate exterior angles.

`6x-36=96`

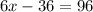
Add 36 on both sides.

`6x-36+36=96+36`

`6x=132`

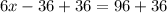
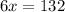
Divide both sides by 6.

`x=(132)/(6)`

`x=22`

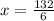
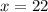
The value of x is 22. Therefore, the correct option is C.

Answer 2

C. 22

Explanation:

Inverse Alternate Interior Angles Theorem sttes that if two lines a and b are cut by transversal f so that the alternate interior angles are congruent, then a║b.

To prove that lines a and b are parallel, equate the measures of angles 96° and (6x-36)°:

`6x-36=96\\ \\6x=96+36\\ \\6x=132\\ \\x=22`