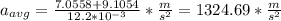Question

To test the quality of a tennis ball, you drop it onto the floor from a height of 4.23 m. It rebounds to a height of 2.54 m. If the ball is in contact with the floor for 12.2 ms, what is its average acceleration during that contact?

Answer 1

`a_(avg)=1324.69*(m)/(s^(2) )`

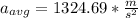
Explanation:

The average acceleration is given by:

`a_(avg)=(\Delta v)/(\Delta t) =(v_(f)-v_(0))/(\Delta t)`

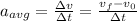
where
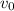`v_(0)` is the velocity of the ball an instant before hitting the ground and
`v_(f)` an instant after.

we can obtain
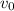`v_(0)` by applying the following formula:

`v_(0)^(2) =v'_(0)^(2) -2*g*\Delta y`

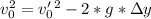
where
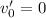`v'_(0)=0` since the ball falls from rest, g is the acceleration of gravity and
`\Delta y=(0-4.23)*m=-4.23*m`

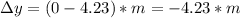
then:

`v_(0)=\sqrt{v'_(0)^(2) -2*g*\Delta y}`

`\pm v_(0)=\sqrt{0^(2) -2*9.8*(-4.23)}`

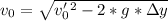
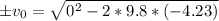
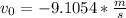`v_(0)=-9.1054*(m)/(s)` (note that here we select the negative result because of the downwards direction of the velocity)

Similarly, we calculate
`v_(f)` with the formula:

`v_(f)^(2) =v'_(f)^(2) -2*g*\Delta y`

where
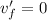`v'_(f)=0` is the velocity at the maximum height, and
`\Delta y=(2.54-0)*m=2.54*m`

then:

`v_(f)=√(2*9.8*2.54)`

`v_(f)=7.0558*(m)/(s)`

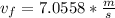
Finally the average acceleration is:

`a_(avg)=(7.0558+9.1054)/(12.2*10^(-3) )*(m)/(s^(2))=1324.69* (m)/(s^(2))`