Answer:
years to maturity:
Step-by-step explanation:
The market value will be the present value of the bons at 9.34% YTM
Present value of the cuopon payment will be an aordinary annuity:

C 40 (1,000 x 8%/2 payment per year
time n (unknow value)
rate 0.0934

Present value of the maturity, which is present value of a lump sum
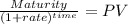
Maturity 1,000.00
time n
rate 0.0467 (rate / 2 as there are 2 payment per year)
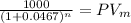
We know that:
PVc + PVm = Market price = 889.83
So we can build this equation:
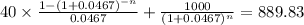
Based on the values we are given, we solve for "n"
First, we work out the annuit y formula:
![(40)/(0.0467) - \frac{40}{0.0467*1.0467^n+ (1000)/((1 + 0.0467)^(n) ) = 889.83\\]()
Then we do common factor:
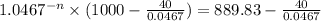
We solve and leave this:
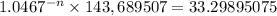

We now apply logarthimic properties to sovle for n
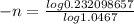
n = 32 These are semiannual payment, so we divide by 2 to convert to year:
32/2 = 16 years