Question

Consider an electron, of charge magnitude e = 1.602 x 10^-19 C and mass me = 9.11 x 10^-31 kg, moving in an electric field with an electric field magnitude E = 9 ✕ 10^2 N/C, similar to what Thana observed in the simulation. Let the length of the plates be L = 50 cm, and the distance between them be d = 20 cm. Find the maximum speed, v, the electron could be moving if it enters the space halfway between and parallel to the two plates to just barely strike one of the plates.

Answer 1

Step-by-step explanation:

Under the given situation , electron will move in two dimension, one along the plates as well as perpendicular to plates on a curved line. Time required to cover the length of plate with given velocity v and travel half the distance between the plate are equal . Initial velocity in in travelling perpendicular to plated will be zero .

Force on the electron is given by the expression

charge on electron x electric field

F = 1.6 x 10⁻¹⁹ x 9 x 10²

=14.4 X 10 ⁻¹⁷ N

Acceleration = force / mass of electron

=
`(14.4*10^(-17))/(9.11*10^(-31))`

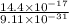
= 1.58 x 10¹⁴ ms⁻²

If time taken to cover half the distance d = 10 cm be t

d = 1\ 2 a t²

t = √2d / a

t =
`\sqrt{(2*10*10^(-2))/(1.58*10^(14))}`

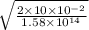
= 3.557 x 10⁻⁸ s

If v be the velocity parallel to plate , distance travelled along plate is 50 x 10⁻² m , time taken is 3.557 x 10⁻⁸

`v = (50*10^(-2))/(3.557*10^(-8))`

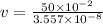
= 14.05 x 10⁶ m /s