Answer:
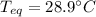
Step-by-step explanation:
Hello!
In this case, since it is observed that hot cadmium is placed in cold water, we can infer that the heat released due to the cooling of cadmium is gained by the water and therefore we can write:
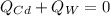
Thus, we insert mass, specific heat and temperatures to obtain:
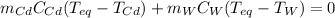
In such a way, since the specific heat of cadmium and water are respectively 0.232 and 4.184 J/(g °C), we can solve for the equilibrium temperature (the final one) as shown below:
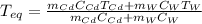
Now, we plug in to obtain:

NOTE: since the density of water is 1g/cc, we infer that 25.00 cc equals 25.00 g.
Best regards!