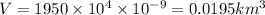Answer:
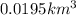
Step-by-step explanation:
1 hectare = 10^4 m^2
Area of pit, A = 75 hectare
convert hectare into m^2
A = 75 x 10^4 m^2
depth, d = 26 m
Volume of earth removed is V.
V = area of pit x depth
V = A x d
V = 75 x 10^4 x 26 = 1950 x 10^4 m^3
Convert cubic metre into cubic km
1 m = 10^-3 km
1 m^3 = 10^-9 km^3
So,