Question

The lengths of two opposite sides of a square are decreased by 40\% while the lengths of the other two sides are increased by 50\% to form a rectangle. By what percent does the square's area decrease?

Answer 1

10% decreased

Explanation:

Answer 2

The area has decreased a 10%.

Explanation:

Lets suppose that the the sides of the square are 1 cm, so the area is 1
`cm^(2)`.

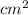so if the square has been reduced 40% in widht, the new widht is 60% of what it was before. that means 0.6cm

then it was increased 50% on height, so the new height is 1.5 cm

The area of a rectangule is (height*widht)

A=(0.6*1.5)

A=0.9 [
`cm^(2)`]

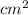The area of 0.9
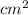`cm^(2)` represent the 90% of the first area, so the area was reduced a 10%. (100%-90%).