Answer: The standard deviation of test scores in the class is not less than 14.1
Explanation:
Let's suppose that the test scores follow a normal distribution. Besides, we have:
a) Standard deviation
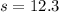
b) Significance level
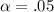
c) n=27
Using a) we can deduce that sample variance
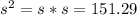 .
.
Since we want to prove if the population variance is less than
 :
:
 (Null hypotesis) :
(Null hypotesis) :
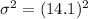
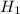 (Alternative hypotesis):
(Alternative hypotesis):

For test this kind of hypotesis (variance in one population) the correct test statistic is
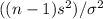 , which under
, which under
 have Chi-square distribution with n-1 degrees of freedom.
have Chi-square distribution with n-1 degrees of freedom.
Calculating the test statistic (
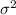 is the value in
is the value in
 ) :
) :
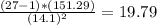
For this hypotesis (left one tailed test) the p-value is
 where M follow a Chi-square distribution with n-1=26 degrees of freedom.You can check in a chi-square table that p-value=0.1986
where M follow a Chi-square distribution with n-1=26 degrees of freedom.You can check in a chi-square table that p-value=0.1986
If
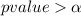 then there is no evidence to statistically reject
then there is no evidence to statistically reject
 . Therefore, the standard deviation of test scores in the class is not less than 14.1 (95% confidence level).
. Therefore, the standard deviation of test scores in the class is not less than 14.1 (95% confidence level).