Question

Assume there are 100 million passenger cars in the United States and the average fuel efficiency is 21 mi/gal of gasoline. If the average distance traveled by each car is 12,600 mi/yr, how much gasoline would be saved per year if average fuel efficiency could be increased to 28 mi/gal?

Answer 1

15 billions gallons are saved a year with the fuel efficiency increased to 28 mi/gal.

Explanation:

This problem can be solved by the use of rules of three.

The first step is find how many gallons are used with the efficiency of 21mi/gal:

1gal are used for 21 miles. How many gal are needed for 12,600 miles. So

1 gal - 21 miles

x gal - 12,600 miles

21x = 12,600

`x = (12,600)/(21)`

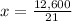
x = 600 gallons

Each passenger uses 600 gallons a year.

There are 100 million passengers a year, so:

1 passenger - 600 gallons

100,000,000 passengers - x gallons

x = 600*100,000,000

x = 60,000,000,000

With the efficiency at 21mi/gal, 60 billion gallons are used a year.

Now with the efficiency at 28 mi/gal

Same logic as above:

1gal - 28 miles

x gal - 12,600 miles

28x = 12,600

`x = (12,600)/(28)`

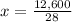
x = 450 gallons

Each passenger uses 450 gallons a year of gasoline.

There are 100 million passengers a year, so:

1 passenger - 450 gallons

100,000,000 passengers - x gallons

x = 450*100,000,000

x = 45,000,000,000

With the efficiency at 28mi/gal, 45 billion gallons are used a year. It means that 60 billion - 45 billion = 15 billions gallons are saved a year with the fuel efficiency increased to 28 mi/gal.