Question

A car traveling east at 40.0 m/s passes a trooper hiding at the roadside. The driver uniformly reduces his speed to 25.0 m/s in 3.50 s. (a) What is the magnitude and direction of the car’s acceleration as it slows down? (b) How far does the car travel in the 3.5-s time period?

Answer 1

Explanation:

It is given that,

Initial speed of the car, u = 40 m/s

Final speed of the car, v = 25 m/s

Time taken, t = 3.5 s

(a) We need to find the magnitude and direction of the car’s acceleration as it slows down. It can be calculated using formula as :

`a=(v-u)/(t)`

`a=(25-40)/(3.5)`

`a=-4.28\ m/s^2`

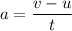
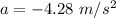
The acceleration is in the opposite direction of motion i.e. west.

(b) Let s is the distance the car travel in the 3.5-s time period. It can be calculated using the third equation of motion as

`s=(v^2-u^2)/(2a)`

`s=(25^2-40^2)/(2* (-4.28))`

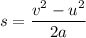
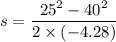
s = 113.9 meters

Hence, this is the required solution.