Question

The temperature inside a certain industrial machine at time t seconds after startup, for 0 < t < 10, is given by h(t) = 42t + 1 – 4t + 2 degrees Celsius. How many seconds after startup is the temperature inside the machine equal to 128 degrees Celsius?

Answer 1

`t= (125)/(38) s`

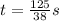
Explanation:

We are given the temperature inside the machine from startup until 10 seconds later, the formula is:

`h(t)= 42 \cdot t +1 - 4\cdot t +2  (degrees \, C)`

We want to know at what time t the temperature inside the machine will be equal to 128 °C.

So we set:

`h(t)=128`

`42\cdot t+1-4\cdot t+2=128`

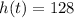
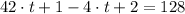
Now, we rearrange the equation to keep terms with t on the left hand side and terms without t on the right hand side

`42\cdot t-4\cdot t=128-1-2`

and we simplify:

`(42-4)\cdot t= 128-2-1= 125`

`38 \cdot t = 125`

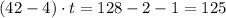
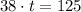
now it's easy to solve for t:

`t=(125)/(38)`

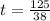
And thus we arrive to the solution.