Question

Mario is constructing a square dart board. It will consist of a smaller square centered in a larger square. The smaller square measures $4$ inches on each side. The ratio of the area of the smaller square to the area of the entire dart board is $\frac 49$. How long is the side of the larger square?

Answer 1

6 inches.

Explanation:

Let a represent side length of larger square.

We know that area of square is square of its side length, so area of the larger square will be
`a^2`.

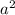The area of smaller square would be
`4^2`.

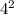We will use proportions to solve our given problem.

`\frac{\text{Area of smaller square}}{\text{Area of larger square}}=(4)/(9)`

`(4^2)/(a^2)=(4)/(9)`

`(16)/(a^2)=(4)/(9)`

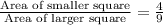
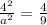
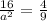
Cross multiply:

`4*a^2=16*9`

`(4*a^2)/(4)=(16*9)/(4)`

`a^2=36`

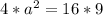
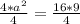
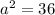
Take square root:

`a=\pm√(36)`

`a=\pm 6`

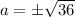
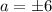
Since the length cannot be negative, therefore, the side length of larger square is 6 inches.