Question

3. The standard equation of a circle (not centered at the origin) with radius r can be derived from its locus definition: The set of all points, P(x, y), that are a given distance, r, from a fixed point C(h, k). How could we use the distance formula and the locus definition to derive the standard equation of a circle?

Answer 1

The equation for circle of radius
`r` centered at the point
`C: (h,k)` is:

`(x-h)^2+(y-k)^2=r^2`

Explanation:

To derive this equation, we need the formula for the distance between two points in the plane. If the point is given by its cartesian coordinates, we can use the Pythagorean theorem to find what the distance between the points is.

Lets say we have two points, one given by cartesian coordinates P:(x,y) and the other given by cartesian coordiantes C:(h,k)

(see diagram)

We can see that

`\Delta x = x-h\\\Delta y = y-k`

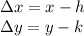
and by the pythagorean theorem we have:

`\Delta s ^2 = \Delta x^2 + \Delta y^2`

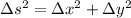
Where
`\Delta s` is the distance between the points

Now, combining the last three equations we find:

`\Delta s ^2 = (x-h)^2 + (y-k)^2`

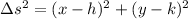
We know that the definition of a circle is the locus of points that are at a distance r from a point called the center of the circle. if we take C:(h,k) as the center of the circle, and
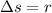`\Delta s  =r` , the last equation becomes:

`r^2 = (x-h)^2 + (y-k)^2`

And that is the result we wanted.