Question

Nickel (II) ions form a complex ion in the presence of ammonia with a formation constant (Kf) of 2.0×10^8:

Ni2+ + 6NH3 ⇌ [Ni(NH3)6]2+
Calculate the molar solubility of NiS in 3.1 M NH3. g

Answer 1

The molar solubility of NiS is 7.7 * 10⁻⁷ M

Step-by-step explanation:

To answer this question, we need to keep in mind two equilibriums.

First, we have the solubilization of NiS:

NiS ⇄ Ni²⁺ + S²⁻ ksp= 3.0 * 10⁻²¹ (we know this from standard tables)

Second, we have the formation of the complex:

Ni²⁺ + 6NH₃ ⇄ [Ni(NH₃)₆]²⁺ kf=2.0 * 10⁻⁸

Combine the two equilibriums and we have

NiS + 6NH₃ ⇄ [Ni(NH₃)₆]²⁺ + S²⁻ K= ksp * kf =6.0* 10⁻¹³=
`([S^(2-)][Ni(NH3)6^(+2)])/([NH3]^(6))`

The molar solubility s is equal to both [Ni(NH₃)₆²⁺] and [S²]

At equilibrium, [NH₃]= 3,1 M - 6s

Thus, if we replace those terms in the formula for K, we're left with:

`(s^(2) )/((3,1-6s)^(2))=6*10^(-13)`

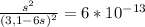
Using an approximation we can ignore the denominator and we have

• s²=6.0 * 10⁻¹³

• s=7.7 * 10⁻⁷