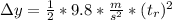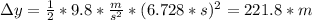Question

You are climbing in the High Sierra where you suddenly find yourself at the edge of a fog-shrouded cliff. To find the height of this cliff, you drop a rock from the top and 7:40 s later hear the sound of it hitting the ground at the foot of the cliff.Ignoring air resistance, how high is the cliff if the speed of sound is 330 m/s?

Answer 1

`\Delta y=221.8*m`

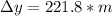
Explanation:

The distance that the rock has traveled to reach the ground (also, the height of the cliff) can be calculated using:

`\Delta y=y_(0)+v_(0)*t+(1)/(2) *g*(t_(r))^(2)`

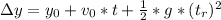
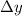`\Delta y` is our incognit

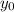`y_(0)` is the starting position of the rock, we'll define it as 0

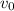`v_(0)` is the starting velocity of the rock, it is 0 since it starts from rest

g is the acceleration of gravity

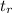`t_(r)` is the time it took the rock reaching the ground

Also, we can model the "movement" of sound as it follows:

`\Delta y=v_(s)*t_(s)`

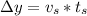
where:

`v_(s)` is the velocity of sound :330m/s

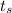`t_(s)` is the time it took the sound travelling to the top of the cliff

using the first and the second equation, and the fact that
`t_(total)=7.4*s=t_(r)+t_(s)` we get to:

`v_(s)*(t_(total)-t_(r))=(1)/(2) *g*(t_(r))^(2)`

`330*(m)/(s) *(7.4*s-t_(r))=(1)/(2) *9.8*(m)/(s^(2) ) *(t_(r))^(2)`

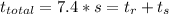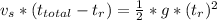
`t_(r)=6.728 *s` (we get to values for tr since the equation is quadratic, the correct one is the positive)

Now that we have
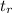`t_(r)` we can use it to calculate
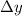`\Delta y` and determine the height of the cliff:

`\Delta y=(1)/(2) *9.8*(m)/(s^(2) ) *(t_(r))^(2)`

`\Delta y=(1)/(2) *9.8*(m)/(s^(2) ) *(6.728*s)^(2)=221.8*m`