Question

A computer lab has two printers. Printer I handles 40% of all the jobs. Its printing time is Exponential with the mean of 2 minutes. Printer II handles the remaining 60% of jobs. Its printing time is Uniform between 0 minutes and 5 minutes. A job was printed in less than 1 minute. What is the probability that it was printed by Printer I?

Answer 1

360

Step-by-step explanation:

Answer 2

Answer: 0.3042

Step-by-step explanation:

Let A and B are the events to that job done by Printer I and Printer II respectively.

Given : P(A)=0.40 P(B)=0.60

Printing time of Printer I is Exponential with the mean of 2 minutes.

i.e. average number of job done in one minute:
`\lambda=(1)/(2)`

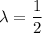
The cumulative distribution function (CDF) for exponential distribution:-

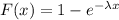`F(x)=1-e^(-\lambda x)`, where
`\lambda` is the mean.

Then, the cumulative distribution function (CDF) for Printer I:-

`P(X|A)=1-e^{-(1)/(2) x}`

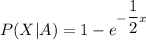
i.e.
`P(X<1|A)=1-e^{-(1)/(2)}`

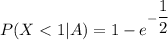
Printing time of Printer II is Uniform between 0 minutes and 5 minutes.

The cumulative distribution function (CDF) for uniform distribution in interval (a,b) :-

`F(x)=(x-a)/(b-a)`

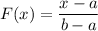
Then,
`P(X|B)=(x-0)/(5-0)=(x)/(5)`

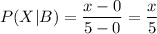
i.e.
`P(X<1|B)=(1)/(5)`

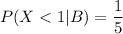
Now, the required probability :-

`\text{P(A}|X<1)=(P(A) P(X<1|A))/(P(A) P(X<1|A)+P(B) P(X<1|B))\\\\=\frac{(0.4)(1-e^{-(1)/(2)})}{(0.4)(1-e^{-(1)/(2)})+(0.6)((1)/(5))}}\\\\=((0.4)(0.3935))/((0.4)(0.3935)+(0.6)(0.6))\\\\=0.304213374565\approx0.3042`

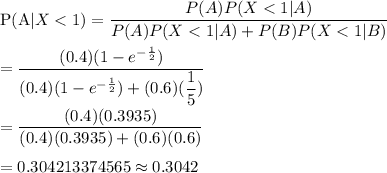
Hence, the required probability = 0.3042