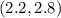Answer:
C is
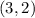
D is
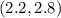
Explanation:
(i) We need to find the coordinate of point C, which divides A(1,4) and B(6,-1) in the ratio 2:3
We know that the coordinate of a point
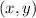 dividing a line segment joining
dividing a line segment joining
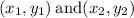 in the ratio m:n is given by
in the ratio m:n is given by
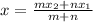 ,
,
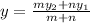
Let the coordinate of point C be
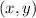
Here, the ratio is 2:3
So,
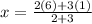
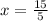


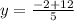
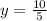
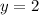
Hence, coordinate of C is
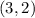
(ii) We need to find the coordinate of point D, which divides A(1,4) and C(3,2) in the ratio 3:2
We know that the coordinate of a point
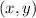 dividing a line segment joining
dividing a line segment joining
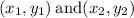 in the ratio m:n is given by
in the ratio m:n is given by
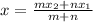 ,
,
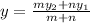
Let the coordinate of point D be

Here, the ratio is 2:3
So,
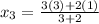


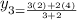
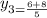
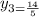
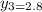
Hence, coordinate of D is