Answer:
a)
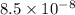 N
N
b)
 m/s
m/s
Step-by-step explanation:
a)
 = radius of the orbit = 0.522 x 10⁻¹⁰ m
= radius of the orbit = 0.522 x 10⁻¹⁰ m
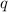 = magnitude of charge on each proton and electron = 1.6 x 10⁻¹⁹ C
= magnitude of charge on each proton and electron = 1.6 x 10⁻¹⁹ C
Using coulomb's law , magnitude of electric force on the particle is given as
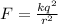
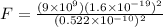
 N
N
b)
 = mass of electron = 9.1 x 10⁻³¹ kg
= mass of electron = 9.1 x 10⁻³¹ kg
 = speed of electron = ?
= speed of electron = ?
Centripetal force is being provided by the electric force, hence
Centripetal force = electric force
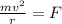
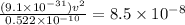
 m/s
m/s