Question

Pizza Paradise offers 4 types of crust, 10 toppings, and 8 kinds of cheese for the mega calzone. How many different mega calzones can be made if a mega calzone contains 3 different toppings and 3 different cheeses

Answer 1

26880 ways

Explanation:

Given

`Crust = 4`

`Toppings = 10`

`Cheese = 8`

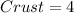
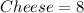
Required

Determine the number of ways 3 toppings and 3 cheese can be selected

The number of crusts to be selected was not stated. So, I'll assume 1 crust to be selected from 4.

This can be done in
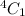`^4C_1` ways

For the toppings:

3 can be selected from 10 in
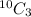`^(10)C_3` ways

For the cheeses:

3 can be selected from 8 in
`^(8)C_3` ways

Total number of selection is:

`Total = ^4C_1 * ^(10)C_3 * ^(8)C_3`

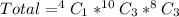
Apply combination formula:

`Total = (4!)/((4-1)!1!) * (10!)/((10-3)!3!) * (8!)/((8-3)!3!)`

`Total = (4!)/(3!1!) * (10!)/(7!3!) * (8!)/(5!3!)`

`Total = (4*3!)/(3!*1) * (10*9*8*7!)/(7!*3*2*1) * (8*7*6*5!)/(5!*3*2*1)`

`Total = (4)/(1) * (10*9*8)/(3*2*1) * (8*7*6)/(3*2*1)`

`Total = 4 * (720)/(6) * (336)/(6)`

`Total = 4 * 120* 56`

`Total = 26880`

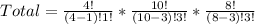
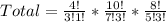
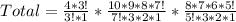
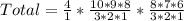
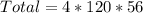
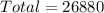
Hence, there are 26880 ways